Anderson’s Bridge

Definition: Anderson’s Bridge is an AC bridge that determines the self-inductance of an inductor in the circuit. This bridge offers highly accurate output and is an advancement of Maxwell’s inductance capacitance bridge.

By Anderson’s bridge, one can measure inductance by utilizing other circuit components like resistors and capacitor. In Maxwell’s bridge, the inductance is calculated by comparing it with a standard variable capacitance.

In this article, we will discuss the construction, theory as well as the mathematical expression to calculate the unknown inductance of a coil connected in the circuit.

Construction of Anderson’s Bridge

The figure below shows the constructional structure of Anderson’s bridge:

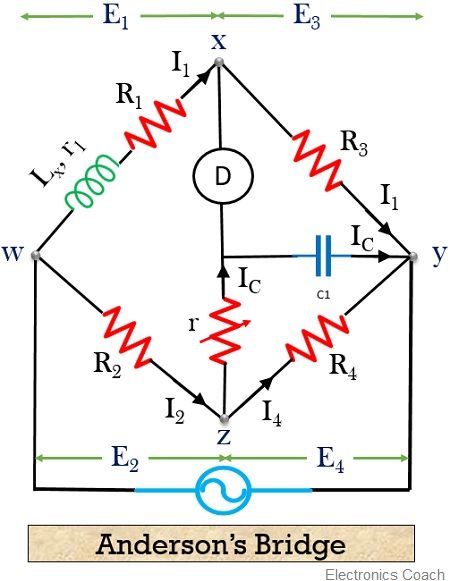
As we can see the bridge has junctions w, x, y and z that jointly forms 4 arms wx, xy, yz, zw.

The unknown inductance is connected in one of the arms along with a variable resistance R₁. While the rest 3 arms of the bridge contain serially connected resistive elements.

Let us move further to understand the theory associated with Anderson’s bridge and how the unknown inductance is precisely calculated.

Theory and Mathematical Expression of Anderson’s Bridge

Here, for the above figure

• L_x is the unknown inductance to be measured,
• r₁ is the resistance offered by the coil,
• R₁ is the external variable resistance connected serially with the unknown inductance,
• r, R₂, R₃, R₄ are the known resistances

and

• C is a fixed capacitor with known capacitance

At the balanced condition, the current through the detector must be 0. So,

I₁ = I₃ ——————– eqⁿ 1

and

I₂ = I_C + I₄  ——————– eqⁿ 2

On equating the drop across R₃ and C, we will get

 

 

So, from the above equation

I_C = I₁ jωC R₃        ——————– eqⁿ 4

Also, the voltage drop across arm wx must be equal to the sum of the voltage drop across yz and zw. So, we get

I₁(R₁ + r₁ + jωL_x) = I₂R₂ + I_C r      ——————– eqⁿ 5

On substituting the value of I_C in the above equation, we get

I₁(R₁ + r₁ + jωL_x) = I₂R₂ + I₁ jωC R₃ r     ——————– eqⁿ 6

On transposing, we get

I₁(R₁ + r₁ + jωL_x – jωC R₃ r) = I₂R₂       ——————– eqⁿ 7

Further, when the voltage across r and C is equated with the voltage at R₄, we will have

 

 

On transposing

 

 

Again substituting the value of I_C in the above equation

 

 

On dividing eqⁿ 7 from eqⁿ 10

 

 

 

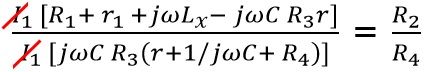
So,

R₁R₄+ r₁R₄ + jω L_x R₄ – jωC R₃R₄ r = jωC R₂R₃ (r + R₄) + R₂R₃

R₁R₄ + jω (L_xR₄ – CR₃R₄ r) = jωCR₂R₃ (r + R₄) + R₂R₃ – r₁R₄

On equating the real part and imaginary part separately from the above equation

Real

R₁R₄ = R₂R₃ – r₁R₄

 

 

 

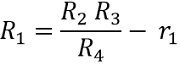
Imaginary Part

 

 

L_xR₄ – C R₃R₄ r = C R₂R₃r + C R₂R₃ R₄

L_xR₄ = C R₃R₄ r + C R₂R₃r + C R₂R₃ R₄

L_xR₄ = C R₃[(R₄ r + R₂ r) + R₂ R₄]

 

 

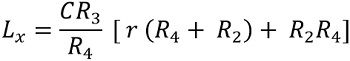
This is the desired equation used to determine the value of unknown inductance. And we can clearly see that the equation is independent of the value of r₁ and R₁.

Phasor Diagram of Anderson’s Bridge

The figure below shows the phasor representation of Anderson’s bridge:

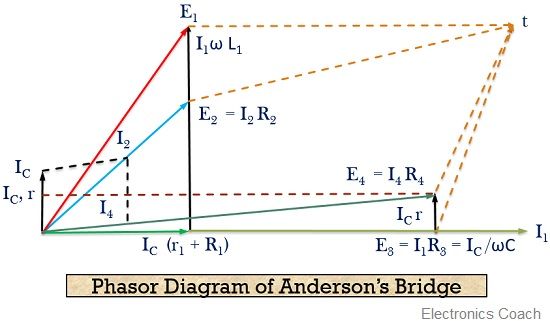
From the bridge circuit, it is clear that the current through arm wx i.e., I₁ and the voltage drop at arm xy i.e., E₃ are in the same phase. This is shown by the horizontal axis in the above figure.

Under the balanced condition, we know the drop across xy and oy must be equal.

The current flowing in arms wx causes the drop I₁(R₁ + r₁) and in phase with arm current I₁. And due to the balanced condition of the bridge, this current flows through arm xy.

Also, the drop across yz i.e., E₄ is equivalent to the sum of drop at oz and oy i.e., I_C/ωC and I_C r respectively.

Thereby showing that the current I₄ will be in phase with the drop across yz i.e., E₄. Also, the phasor summation of drop across wx and xy or drop across yz and zw provides the supply voltage.

Advantages of Anderson’s Bridge

• The bridge is composed of a fixed capacitor that makes the determination of inductance quite easy.
• Also, one can find an accurate value of capacitance in accordance with the inductance.
• It is quite simple to find the balance point in Anderson’s bridge.

Disadvantages of Anderson’s Bridge

• The factor of complexity is highly associated with this bridge due to the presence of more components.
• Due to the existence of an extra junction O, the shielding of the bridge becomes a difficult task.

This is all about Anderson’s bridge inductance evaluation and phasor diagram.