Time Response of First Order System

The type of system having ‘1’ as the maximum power of ‘s’ in the denominator of the transfer function of the control system is known as the first-order system. Thus, we can say, that the order of the system is specified by the highest power of s.

Basically the order of the system shows information regarding the closed-loop poles of the system. Thus for the first-order system, we can say that there will be one closed-loop pole.

Here we can see the block diagram of unity negative feedback first order control system:

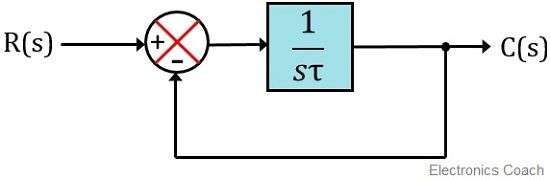
Here in this article, we are about to discuss the time response of the first-order control system. Now the question arises-

What is time response?

The time response of the system is defined as the output of the system obtained by providing specific input to the system, where both input and output must be the function of time.

This means that the time response of the system provides an idea about the variation in output when a certain input is provided with respect to time.

Basically the time response of the system is composed of steady-state response and transient response and is given as:

The transient response represents the fluctuation in the output of the system on applying input before achieving the final value.

While steady-state response represents the finally achieved output of the system.

Sometimes the finally achieved value shows variation from that of the desired value. Thus the difference of desired value and achieved value shows the steady-state error of the system, represented by e_ss.

The closed-loop transfer function of the system is given as:

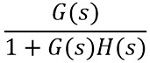
For unity negative feedback system, the characteristic equation gives the poles of the system

So, in general form, the first order equation will be:

Thus the closed-loop pole will be:

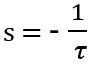
Time Response of First-Order System

We know that to determine the response of the system, some input must be provided to it. So, here we will consider different inputs and will see the response of each input on the first-order control system.

For unit step signal as input

Since the open-loop gain of the first-order system is given as:

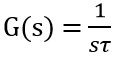
We know that for a unit step input

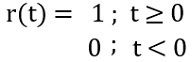
Thus

Taking the Laplace transform of r(t). So,

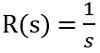
We know that the closed-loop transfer function is given as:

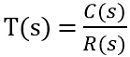
: C(s) is the controlled output and R(s) is the reference input

Since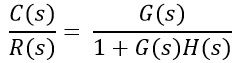

On substituting the open-loop gain, the above equation: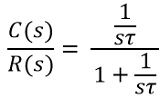

On simplifying,

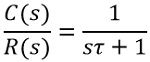
Therefore,

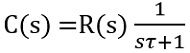
As we have already determined, R(s), for unit step unit. Hence substituting the value of R(s), we will have

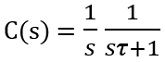
On solving the partial fraction of the above equation,

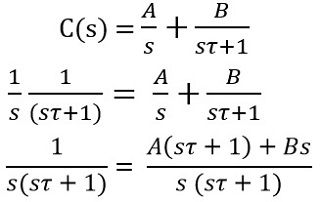
On simplifying,

On equating constant terms

Further substituting A = 1 in the general equation

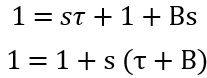
Now, equating the coefficient of s, we will have

Therefore,

On substituting the values of A and B, we will have

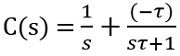
Further,

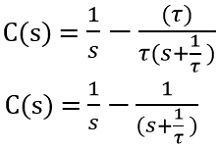Now, taking inverse Laplace transform of the above equation,

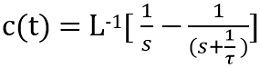
Since,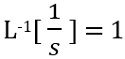

While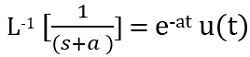

So,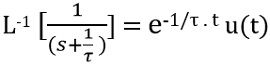

Thus,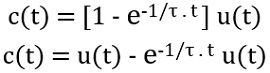

Here u(t) represents the steady-state response while the other term is the transient response of the first-order control system.

The response of the unit step signal is given as:

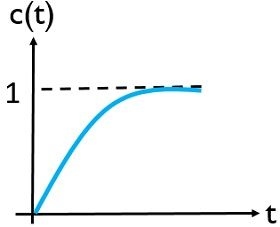
For unit ramp signal as input

We know the unit ramp signal is mathematically expressed as:

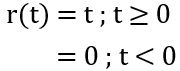
Therefore

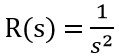
The open-loop gain of the first-order control system is

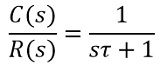
Therefore,

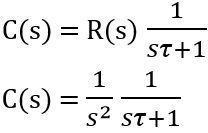
Further on solving

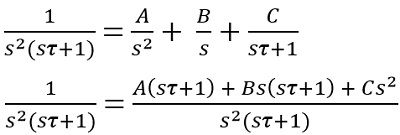
On simplifying

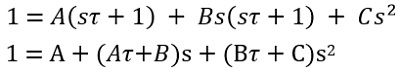
On equating the constant term

On equating the coefficient of s after substituting the value of A

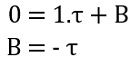
Further equating the coefficient of s₂

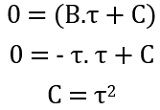
So, on substituting the values of all the values in the general equation for unit ramp signal

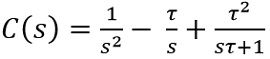
Therefore,

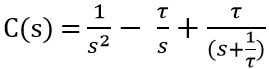
Applying the inverse Laplace transform in the above equation

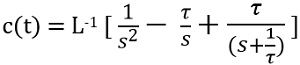
So, we will have

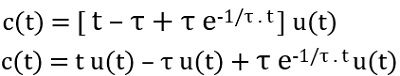
Here

represents the steady-state response while

shows the transient response of the first-order system with unit ramp unit.

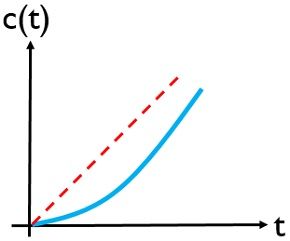
The unit ramp response is:

For unit impulse signal as input

The unit impulse input in the time domain is given as:

Taking Laplace transform

Since the closed-loop transfer function is

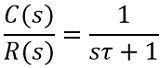
Substituting the value of R(s)

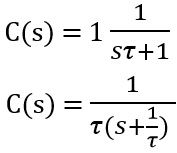
Thus

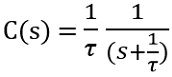
Taking inverse Laplace transform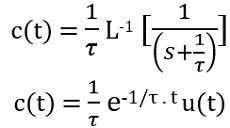

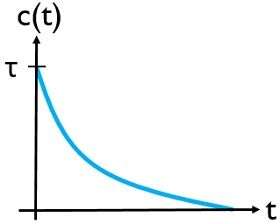
This is the time response of the first-order system with unit impulse input.

The unit impulse response is given as:

For unit parabolic signal as input

The reference input in the time domain is given as

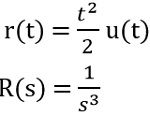
As the open-loop gain is

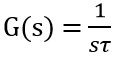
The transfer function is given as

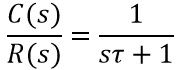
So,

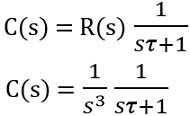
On solving the partial fraction

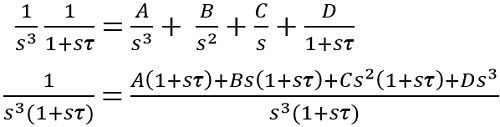
So, on simplifying

On comparing the constant

On comparing the coefficient of s

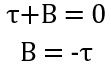
On comparing the coefficient of s²

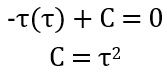
On comparing the coefficient of s³

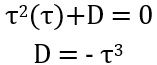
On substituting the values, we will get

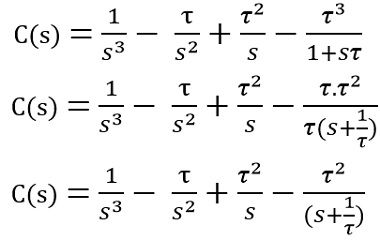
On applying the inverse Laplace transform, we will have

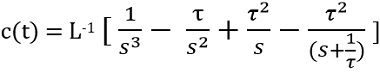
Therefore,

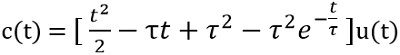
Here,

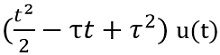
represents the steady-state response and

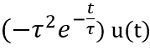
is the transient response.

Generally, for the time domain analysis of first-order control systems, step signals are majorly used as input. This is so because the response of ramp and parabolic input signals in the case of the first-order system increases even with infinite time.

However, as step and impulse functions provide stable responses thus are majorly used. But due to the absence of a steady-state term in the impulse response of the first-order system, step signals are mostly preferred.